When to Use the Ratio Test
The test was first published by Jean le Rond dAlembert and is sometimes known as dAlemberts ratio test or as the Cauchy ratio test. As you might expect the Ratio Test thus gives us information about whether the series a n converges absolutely.

Solved Use The Ratio Test To Decide If The Series Converges Chegg Com
Pearson was chosen because it uses a variable with a ratio data scale in the mini-research example.

. The Ratio Test can be used on any series but unfortunately will not always yield a conclusive answer as to whether a series will converge absolutely or diverge. Decision diagram for the ratio test. The ratio test is similar to the limit comparison test but is only used when the series to be compared against equals 1.
Get step-by-step solutions from expert tutors as fast as 15-30 minutes. 4 n n 3. The ratio test looks at the ratio of a general term of a series to the immediately preceding term.
00 Σ -1 6k ²k 2kk 4 k 1 Select the correct choice below and fill in the answer box within your choice. A If ρ 1 the series P a n converges. B If ρ 1 the series P a n diverges.
If the ratio test does not determine the convergence or divergence of the series then resort to another test. The drawback is that the test sometimes does not provide any information regarding convergence. My doubt is that since the series alternates we should use alternating series test.
If the ratio equals 1 then the series may be divergent conditionally convergent or absolutely convergent. If L 1 then the series. Notice that the Ratio Test considers the ratio of the absolute values of the terms.
A n 1 4 n n 3 n. The ratio test is particularly useful for series whose terms contain factorials or exponentials where the ratio of terms simplifies the expression. The test is inconclusive if L1.
The ratio test works by looking only at the nature of the series youre trying to figure out as opposed to the tests which compare the test youre investigating to a known benchmark series. Therefore we need to evaluate we have therefore. While the Ratio Test is good to use with factorials since there is that lovely cancellation of terms of factorials when you look at ratios the Root Test is best used when there are terms to the n t h power with no factorials.
There are examples with L 1 that converge absolutely examples that converge conditionally and examples that diverge. 3 L1 the series either converges or diverges. The series converges absolutely by the Ratio Test because r OB.
Up to 10 cash back We will use the ratio test to check the convergence of the series. Calculus Tests of Convergence Divergence Ratio Test for Convergence of an Infinite Series 1 Answer Wataru Oct 19 2014 It is not always clear-cut but if a series contains exponential functions orand factorials then Ratio Test is probably a good way to go. Theorem Let a n be a positive sequence with lim n a n1 a n ρ exists.
Use the Ratio Test to determine if the series converges or diverges. Guys im collabing with a youtuber to make a lil horror video with blender animation and cool ass effectsthis was to test aspect ratio by using cinema ratio. C If ρ 1 the test is inconclusive.
The drawback is that the test sometimes does not provide any information regarding convergence. Next for the choice of the correlation coefficient you select Pearson. Sum_ n1 inftyfrac 4nn3 n n 1 n.
The ratio test is used most often when our series includes a factorial or something raised to the nth power. The ratio test is convenient because it does not require us to find a comparative series. The ratio test Remark.
You dont need either test. 70 1 lim converges diverges. If the ratio is less than 1 the series converges absolutely.
Test the absoluteconditional convergence of the series n 1 1 n 2 n 7 5 n 2 n. Move the sales Y and advertising cost X variables into the variables box. Use the Ratio Test or the Root Test to determine whether the following series converges absolutely or diverges.
I am confused whether to use alternating series test or the ratio test. The Ratio Test states. The ratio test states that.
Use the Ratio Test to determine the convergence or divergence of the series. The series is geometric and. Once we find a value for L the ratio test tells us that the series converges absolutely if L1 or if L is infinite.
The ratio test is a way to determine whether a series converges or not. The ratio test compares the series P a n with an. The ratio test is convenient because it does not require us to find a comparative series.
In this section we will discuss using the Ratio Test to determine if an infinite series converges absolutely or diverges. I hope that this was helpful. Then if 1 L1 the series diverges.
A proof of the Ratio Test is also given. This calculus 2 video tutorial provides a basic introduction into the ratio test. If you need to use coor -c enter INFINITY or INFINITY respectively -1int 1315.
The ratio test is particularly useful for series whose terms contain factorials or exponentials where the ratio of terms simplifies the expression. Type an exact answer in simplified form O A. If the Ratio Test is inconclusive determine the convergence or divergence of the series using other methods.
If the ratio is more than 1 the series diverges. To start correlation analysis in SPSS you click Analyze click correlate and click Bivariate. The usual form of the test makes use of the limit.
Examples include the ratio test with factorials exponents fractions and. And therefore This means that. Check connvergennce of series usinng the ratio test step-by-step.
When I used ratio test then it converges.
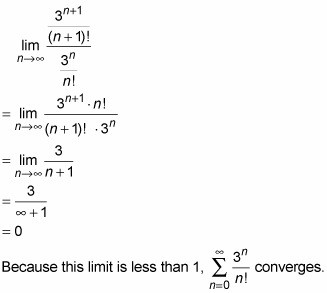
Using The Ratio Test To Determine Whether A Series Converges Dummies

Sequences And Series When To Use The Root Test Is This Not A Good Situation To Use It Mathematics Stack Exchange
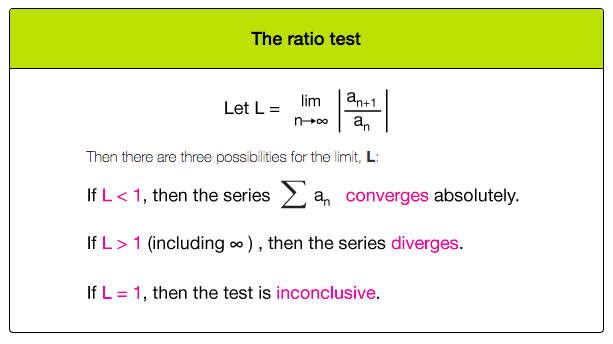
Ratio Test This Test Is Good For Factorials By Solomon Xie Calculus Basics Medium
No comments for "When to Use the Ratio Test"
Post a Comment